The easiest way is to blow off the rice when there's only 1 or 2 squared used. He'll have to restart, wasting precious turns
AnarchyChess
Holy hell
Other chess communities:
[email protected]
[email protected]
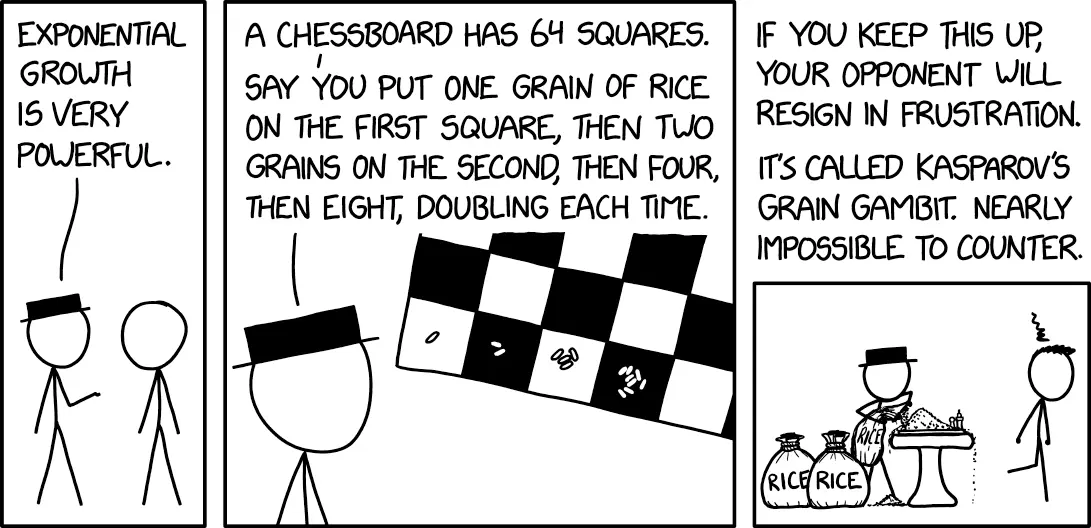
Exponential growth looks fast but if you adopt an even faster strategy like the tree sequence you should be able to outpace your opponent.
It is a bit of a pay to win mechanic, so I hope they fix both these exploits in the next patch.
TREE(TREE) of rice grains is how we get a black hole. Good job, c/anarchychess
I don't think you need nearly that many rice grains.
The only winning move is to cook a nice curry, because plain rice is just boring.
oh my god not the rice
Wait my rectal cheating device (rcd) is receiving something: Many a man, many a mind but every rice husk contains a grain. Must be picking up interference..
I threw this at ChatGPT, it was entertaining.
Q: If you had a chessboard with dimensions comparable to the standard world chess tournament boards, and placed a grain of rice on a square, and then moved to the next square and doubled the amount of rice on that square that you used in the previous one, and kept doing this pattern of picking a square and placing twice as many grains as you did on the last one, approximately how many squares could you fill before the rice starts to overflow into other spaces?
A: Let's analyze the problem step-by-step to determine how many squares can be filled before the rice starts to overflow.
-
Understanding the problem: You start with 1 grain of rice on the first square of a chessboard. On each subsequent square, you double the number of grains of rice from the previous square. This means the number of grains on the ( n )-th square is ( 2^{n-1} ).
-
Capacity and overflow: We need to find the point at which the total amount of rice becomes excessively large, potentially exceeding the board's capacity. This problem is fundamentally a geometric progression and exponential growth problem.
-
Chessboard specifics: A standard chessboard has 64 squares.
-
Exponential growth: Let's calculate the number of grains of rice on a few key squares to understand the rapid growth:
- 1st square: ( 2^0 = 1 ) grain
- 2nd square: ( 2^1 = 2 ) grains
- 3rd square: ( 2^2 = 4 ) grains
- 4th square: ( 2^3 = 8 ) grains
- 5th square: ( 2^4 = 16 ) grains
- ...
- 10th square: ( 2^9 = 512 ) grains
- 20th square: ( 2^{19} = 524,288 ) grains
- 30th square: ( 2^{29} = 536,870,912 ) grains
- 40th square: ( 2^{39} = 549,755,813,888 ) grains
- 50th square: ( 2^{49} = 562,949,953,421,312 ) grains
- 60th square: ( 2^{59} = 576,460,752,303,423,488 ) grains
-
Total grains on the board: The total number of grains of rice after filling all 64 squares is the sum of the geometric series: [ S = 2^0 + 2^1 + 2^2 + \ldots + 2^{63} ] The sum of this series can be calculated as: [ S = 2^{64} - 1 ] This is a massive number: [ 2^{64} - 1 \approx 18.45 \times 10^{18} \text{ grains} ]
-
Practical limitations: In reality, the amount of rice that can fit on a square or even within the confines of a board or room will quickly become unrealistic after a relatively small number of squares. For example:
- The number of grains of rice fitting on a square inch might be around 1,000 grains.
- If each grain is about 0.01 cubic inch, a cubic foot would hold approximately 12,000 grains.
Given the calculations above, the exact square where the rice starts to overflow would depend heavily on the physical capacity of the square, which we've assumed to be quite large in this hypothetical.
- Conclusion: Practically speaking, the rice would start to overflow the physical space far earlier than the 64th square. Assuming a generous physical capacity of a few thousand grains per square:
- By around the 20th to 30th square, the exponential growth would likely have already led to a number of grains that exceed typical containment capabilities.
Therefore, if we were to realistically consider overflow within a standard physical context, you might see overflow happening anywhere from the 20th to 30th squares, given the doubling nature of the sequence.
As often told in online circles, with violence.
I counter with The Plow opening. It‘s guaranteed to shovel all that nastiness out of the way.
New opening just dropped.
only way to combat a gambit is with sentinels
Wait until the piles of rice get big and ask that the opponent be disqualified for being not in their seat while they're getting the next truckload, or cheating with outside help with rice deliveries.