this post was submitted on 12 Jan 2024
1254 points (99.1% liked)
tumblr
4167 readers
734 users here now
Welcome to /c/tumblr, a place for all your tumblr screenshots and news.
Our Rules:
-
Keep it civil. We're all people here. Be respectful to one another.
-
No sexism, racism, homophobia, transphobia or any other flavor of bigotry. I should not need to explain this one.
-
Must be tumblr related. This one is kind of a given.
-
Try not to repost anything posted within the past month. Beyond that, go for it. Not everyone is on every site all the time.
-
No unnecessary negativity. Just because you don't like a thing doesn't mean that you need to spend the entire comment section complaining about said thing. Just downvote and move on.
Sister Communities:
-
/c/[email protected] - Star Trek chat, memes and shitposts
-
/c/[email protected] - General memes
founded 2 years ago
MODERATORS
you are viewing a single comment's thread
view the rest of the comments
view the rest of the comments
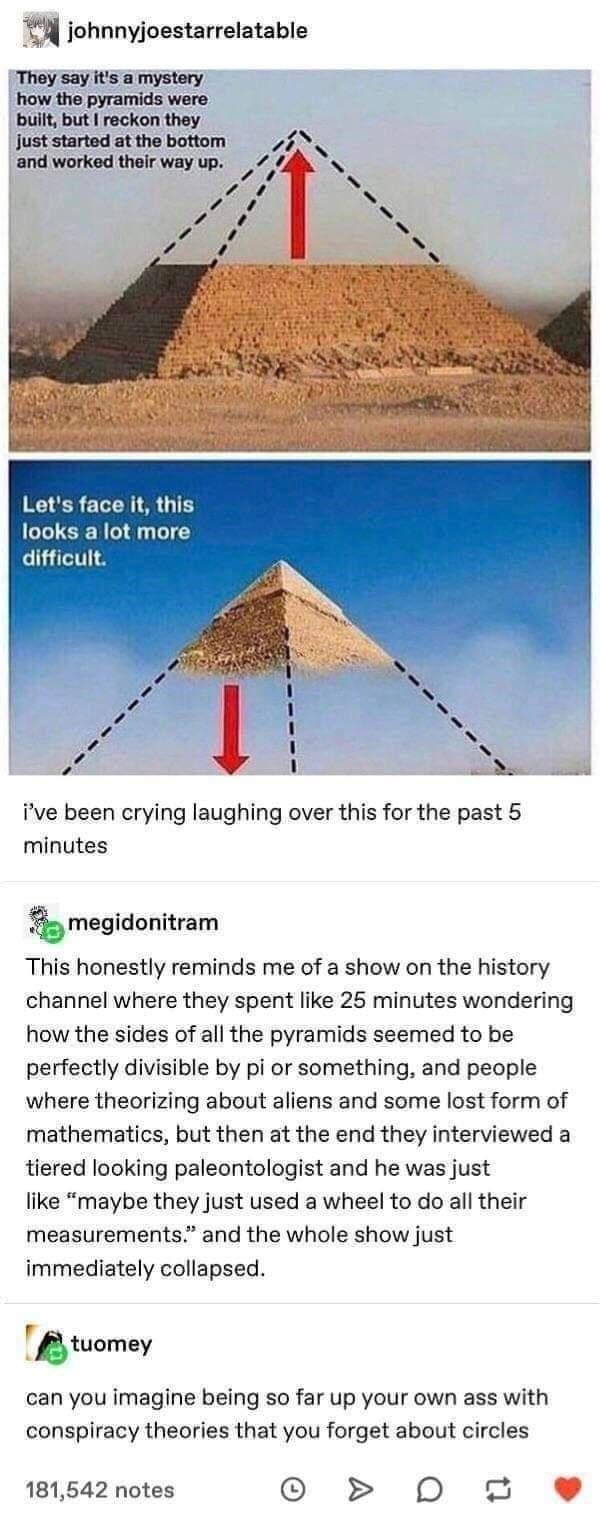
You are right, maybe, in that people are simplifying it too much.
Pi is the ratio of the circumference of a circle to its radius. Measure out lengths using a unit circle and your lengths will be a multiple of the circumference of the wheel used.
But what does it mean that pi pops up in pyramid construction? I think they mean it is the perimeter divided by the height of the great pyramid, which is 2pi. But how would measuring the perimeter in wheels cause that to just fall out? It wouldn't. That would depend on the slope of the sides.
So where did pi come from? From the ratio of their standard units of measure, cubits, palms, and fingers most likely. They would have measured "rise over run" using standard units of measure. Most pyramids slopes end up being a ratio of these units, but not all of them approximate pi.
Source: my recollection from my math history courses for my undergrad math degree.
If you know the ancient unit of length, make a circle with the radius of that length, The various lengths of a line of stones on a side will introduce Pi. The slope would be determined by the height of the blocks and the number of revolutions marking the next run of stones. It is a simple and elegant geometry.
I don't know that I follow your illustration, but let me try an alternate explanation for my point.
One way pi appears is by taking the perimeter of the great pyramid and dividing by its height to get 2Pi. We can write this as
4L/h ~= 2Pi
2L/Pi ~= h
The great pyramid has a height of 280 cubits, and a base of 440. From this, using right triangles and Pythagorean identities we can find the long side and all angles.
Specifically, we look at the slope of the outer face, which we can express as rise over run. In our case, 280/220 = 1.27 or as 51.843 degrees.
As long as that ratio of rise over run holds you will preserve the above ratio with pi. You can fix that slope and vary either the base or the height, and the other value is predetermined. So how did the rise and run ratio come up? Not every pyramid had this relationship, and there is an infinite number of unit length combinations that wouldn't give rise to this ratio.
So let's look at the old units, in particular, the palm.
1 palm = 4 digits.
4 palms = 16 digits.
5 palms = 20 digits.
If for every 5 palms up, the block is 4 palms deep, you get the ratio of 1.25.
That gets us within the 4% agreement with our starting ratio.
Again, you could choose a unit length, and have the rise be 3 up for every 2 palms deep, and you now wouldn't have this ratio. There are an infinite number of ways to construct a pyramid with a unit measure that doesn't.
I hope that makes sense. Have a good day.
We are talking about two separate things. You are correct in what you are writing. We both agree on this. What the article was referencing was the lengths of the various sides. It did not speak about height at any time. There is no rise, only runs.
The runs 'mysteriously' used a unit of length that used a multiple of their unit of measure * Pi. The Archeologist commented that the likely reason was that their wheel was their unit as part of the diameter or radius, hence introducing Pi as part of the measure of the sides. That's the SMH 'Doh' of the comment and the humor.
It is the same humor that poked fun at the engineering graduate who was hired by Edison. He was assigned the task of finding the volume of a new light bulb. After days of work and a long formula measuring it, he brought his results to Edison. Edison said that his was too complicated for him to figure. Edison filled the bulb with water and poured it into a measured beaker.
I have no idea if the Edison story is true, but always remembered it as a young engineering student, to not overcomplicate results. I worked with expert craftsmen who would chew me up and spit me out, if I were caught that way.
Have a good day, also.
That makes sense, I honestly wasn't thinking in terms of the comment on the meme. Thanks for the clarification and have a great day!