Science Memes

11399 readers

1054 users here now

Welcome to c/science_memes @ Mander.xyz!

A place for majestic STEMLORD peacocking, as well as memes about the realities of working in a lab.

Rules

Don't throw mud. Behave like an intellectual and remember the human.
Keep it rooted (on topic).
No spam.
Infographics welcome, get schooled.

This is a science community. We use the Dawkins definition of meme.

Research Committee

[email protected]

Other Mander Communities

Science and Research

Biology and Life Sciences

Physical Sciences

Humanities and Social Sciences

Practical and Applied Sciences

Memes

Miscellaneous

founded 2 years ago

MODERATORS

[email protected]

197

Tensors (mander.xyz)

submitted 3 months ago by [email protected] to c/[email protected]

16 comments fedilink hide all child comments

you are viewing a single comment's thread
view the rest of the comments

[–] [email protected] 6 points 3 months ago* (last edited 3 months ago) (1 children)

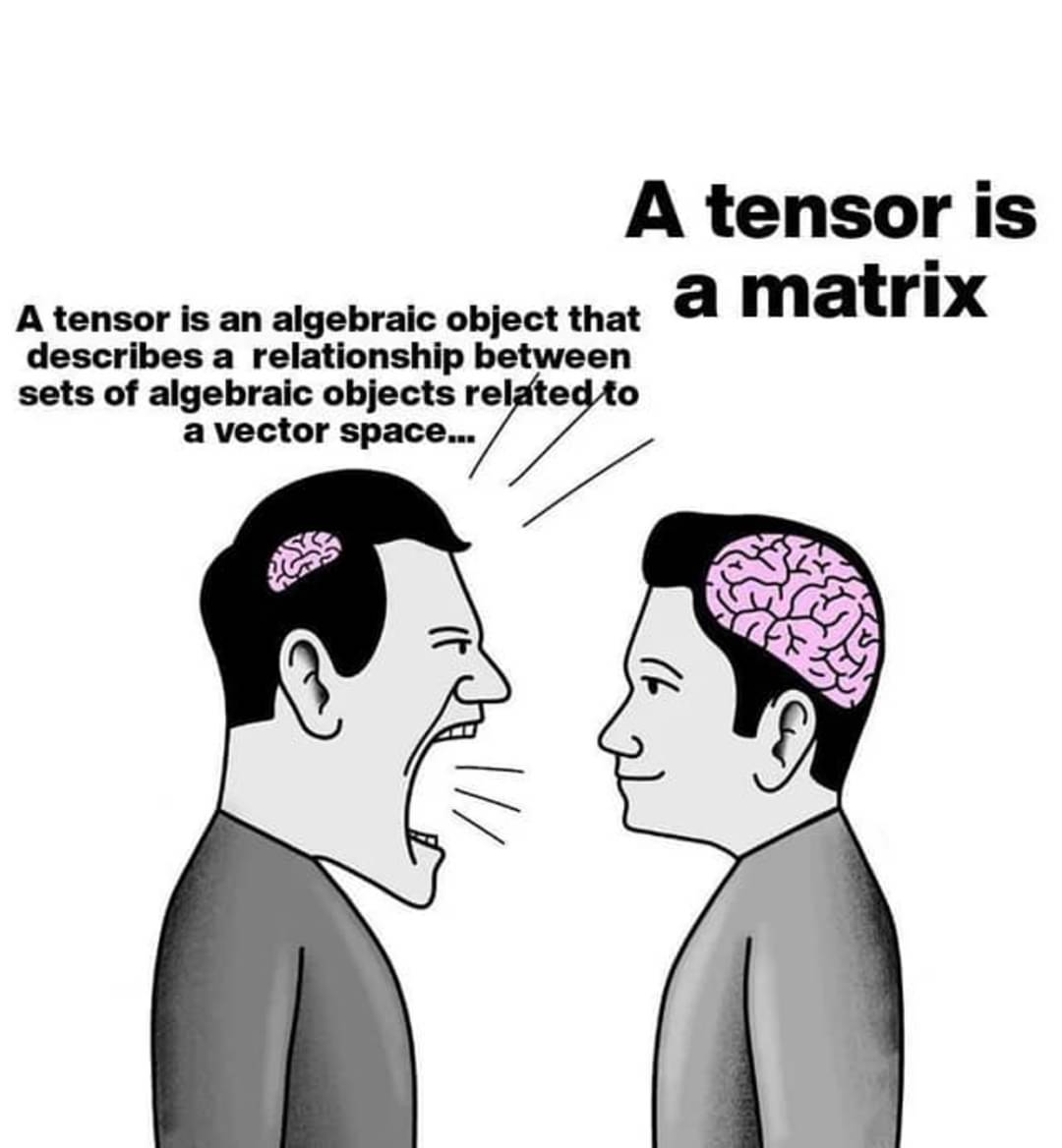
Square matrices are linear endomorphisms. They are isomorphic to (1,1) tensors but not any other rank of tensors.

[–] [email protected] 3 points 3 months ago

a tensor is a multi-linear map V × ... × V × V^^ × ... × V^^ → F, and a multi-linear map V × ... × V × V^^ × ... × V^^ → F is the same as a linear map V ⊗ ... ⊗ V ⊗ V^^ ⊗ ... ⊗ V^^ → F. and a linear map is ""the same thing as"" a matrix. so in this way, you can associate matrices to tensors. (but the matrices are formed in the tensor space V ⊗ ... ⊗ V ⊗ V^^ ⊗ ... ⊗ V^^, not in the vector space V.)